2.5 - Bidirectional reflection
So far we have seen that the visual perception of colour is related to the differential absorption/reflection of radiation by an object in the different regions of the electromagnetic spectrum. But why do we perceive different intensities of light? What is the physical explanation for the creation of patterns of light and dark?
The concept of bidirectional reflection helps explain why objects look different under different lighting conditions and viewing angles, and it is crucial for remote sensing image interpretation. The term Bidirectional Reflectance Distribution Function (BRDF) quantitatively describes how light is reflected at different angles of incidence and reflection, considering the physical properties of the material and the roughness of the surface. The key elements of Bidirectional reflection are:
- Angle of incidence: This is the angle at which incoming light rays strikes the surface.
- Angle of Reflection: This is the angle at which light rays leave the surface after reflection. In a perfect mirror-like (specular) reflection, the angle of reflection is equal to the angle of incidence. Surfaces that are not perfectly smooth scatter light in different directions.
- Reflectivity of the Surface: Surfaces differ in the way they reflect light. Some are specular (like a mirror), some are diffuse (like matte paint), and many are a combination of both. These characteristics are captured in the BRDF.
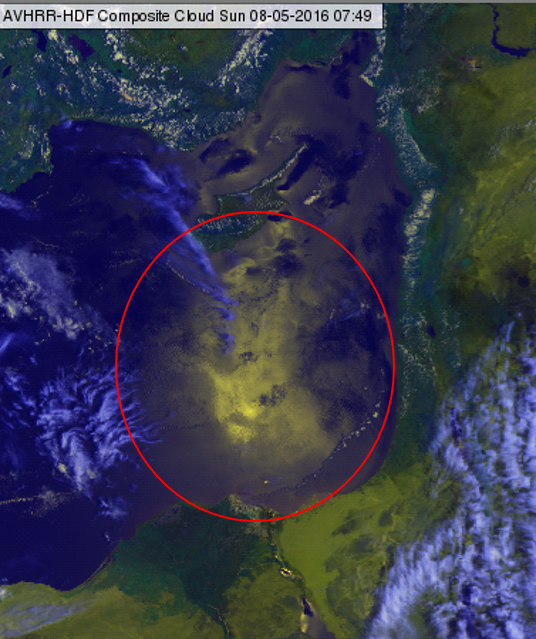
Sun glint (Figure 2.11) is the result of specular reflection, where light rays are reflected at an angle equal to the angle of incidence. This type of reflection is different from diffuse reflection, which scatters light in many directions and is typical for rough surfaces.
Figure 2.11: Sun glint over the east Mediterranean on 8 May 2016 at 07:49 UTC
2.5.1 - BRFDs
The measured surface reflectance depends on the configuration: sun position - observation geometry.
First think of a geostationary satellite, located at fixed position above the Earth. For each individual pixel only the Sun geometry varies from slot to slot.
For the case of polar orbiters, (normally sun-synchronous) it is mainly the satellite observation angles that change between observation slots of the same region.
This means that reflectance values measured with geostationary or polar satellites need to be corrected for directional effects. Such corrections may be easily performed if the Bidirectional Reflectance Distribution Functions (BRDF) are known. The BRDF model describes reflection behavior for all possible combinations of sun-viewing geometries. The characteristics of the BRDF will determine what "type" of material the viewer thinks the displayed object is composed of.
Among the several Bi-directional Reflectance Distribution Functions proposed by different authors, the one by Roujean et al. (1992) has been extensively used for vegetation parameters estimations:
ρ (θs, θv, φ) = K0 + K1 x F1(θs, θv, φ) + K2 x F2(θs, θv, φ)
F1 and F2 are functions, called kernels, which only depend on the angular configuration of aquisition:
- θs is the sun zenith angle;
- θv is the satellite zenith angle
- φ is the relative azimuth angle between sun and satellite
The K coefficients quantify the respective contribution of each kernel to the whole BRDF and are derived by model inversion.
- K0 corresponds to isotropic reflectance, i.e. reflectance values directionally normalised to reference illumination and observation zenith angles of 0°. This geometry leads to a minimum of the shadow proportion (hotspot geometry);
- K1 and K2 represent angular distribution related to geometric (caused by diffuse reflectors) and volumetric (caused by volume scatter reflectors) surface scattering processes.
The isotropic, geometric and volumetric components of surface reflectance are intrinsically linked to the amount and state of vegetation, canopy structure and to the leaf and soil optical properties, as you will see in more detail in chapter 5.